下面是为大家准备的2013年6月30日—7月12日的4道SAT考试数学选择题练习题。同学们在备考SAT数学的过程中，一定要加强习题的练习，熟练到能充分理解题意，争取得到满分。
1.
,
,
,
, ... The first four terms of a sequence are shown above. Which of the following could be the formula that gives the
th term of this sequence for all positive integers
?
(A)
(B)
(C)
(D)
(E)
2.
If 
is a number from Column 
and 
is a number from Column 
in the table above, how many different values are possible for 
?
(A) Nine
(B) Eight
(C) Seven
(D) Six
(E) Five
3.If
, what is the value of
?
(A)
(B)
(C)
(D)
(E)
4.
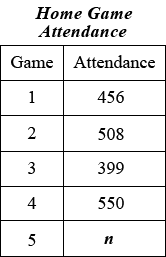
The table above shows the attendance at the home games of the Central High School football team. If the median attendance for the five games was 
, and no two games had the same attendance, what is the greatest possible value for 
?
(A) 
(B) 
(C) 
(D) 
(E) 
5.Triangle 
is isosceles, and the measure of angle 
is 
. What must be the measure of another angle of this triangle?
(A) 
(B) 
(C) 
(D) 
(E) It cannot be determined from the information given.
6.
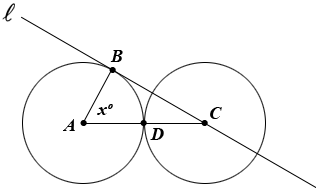
In the figure above, the circle with center 
and the circle with center 
are tangent at point 
. If the circles each have radius 
, and if line 
is tangent to the circle with center 
at point 
, what is the value of 
?
(A) 
(B) 
(C) 
(D) 
(E) It cannot be determined from the information given.
参考答案及解析：
1.The correct answer is E
Explanation
If a formula gives the
th term of the sequence for all positive integers
, it must give the
th term of the sequence for
. If
, then
and
, but the first term of the sequence is
, so the general formula cannot be
or
. Also, if
, then
and
, but the third term of the sequence is
, so the general formula cannot be
or
. However, for
, the values of
are
,
,
,
, respectively. Therefore, the general formula for this sequence could be
.
2.The correct answer is E
Explanation
First let 
equal the first value from Column 
, that is, 
. Then add 
to each of the three values of 
in Column 
as follows: 
, 
, and 
. Repeat this process for 
, for which the possible values of 
are 
, 
, and 
; and repeat it again for 
, for which the possible values of 
are 
, 
, and 
. If you compare these nine values, you see that 
, 
, 
, 
, and 
are the onlydifferent possible values of the sums 
; therefore, there are five different values possible for 
.
3.The correct answer is D
Explanation
Both 
and 
. The equation 
cannot be solved for 
because there are two unknowns. The value of 
can be found by solving the equation 
for . It follows that must equal 
. The value of can now be substituted into the equation 
, giving 
. Therefore, 
must equal 
.
4.The correct answer is B
Explanation
The median of five numbers can be found by putting the numbers in increasing order and picking the middle, or third, number from the list. Putting the four numbers you know in increasing order produces the following list:
Thus, if 
is less than or equal to 
, the list of the numbers in increasing order will be
In either case, the median will be 
. However, if 
is greater than 
, the list of numbers in increasing order will be
or 
or 
In these cases, the median will be either 
or 
. Since you know that the median is 
, it must be true that 
is less than or equal to 
. Also, since no two games had the same attendance, the number 
cannot be equal to 
. Therefore, the greatest possible value of 
is 
.
5.The correct answer is E
Explanation
Triangle
is isosceles, and the measure of angle
is
. Let the measure of angle
be
, and let the measure of angle
be
. The sum of the measures of the angles of a triangle is
, so
. An isosceles triangle has two angles of equal measure, so
, or
, or
.
If
, then
, so
, and the measures of the angles of the triangle are
,
and
.
If
, then
, so
, and the measures of the angles of the triangle are
,
and
.
If
, then
, so
, and
. In this case, the measures of the angles of the triangle are
,
and
.
Hence if the measure of angle
is
, the possible measures of angles
and
, respectively, are
and
;
and
; and
and
. Therefore, it is not possible to determine what must be the measure of another angle of this triangle.
6.The correct answer is B
Explanation
The circles each have radius 
, so 
. Since the circles are tangent at point 
, segment 
contains 
and 
. Also, 
and 
are perpendicular because a line tangent to a circle forms a right angle with the radius at the point of tangency. Therefore, 
is a right triangle with hypotenuse 
and side 
of length 
. A right triangle with one side of length one-half that of its hypotenuse is a 
triangle. The 
angle is opposite side 
, so 
.
以上就是关于6道SAT考试数学选择题练习题及其答案和解析，非常详细。同学们在备考阶段，一定要加强习题的练习以及题意的理解，争取SAT数学满分。
